TS Polycet (Polytechnic) 2020 Previous Question Paper with Answers And Model Papers With Complete Analysis

Q) log_xb – log_xa = log_xc – log_xb
∴ ac =
A) a²
B) b²
C) c²
D) None

Q) A = {P, O, L, Y, T, E, C, H, N, 1, Q}, B = {P, O, L, Y, C, E, T, 2020}, B – A =
A) {20}
B) {2020}
C) {40}
D) None

Q) If (5, 2) is the solution of 2x + 5y = 20, ax -by = 0, then (a, b) =
A) (2, 5)
B) (5, 2)
C) (-2, 5)
D) (-5, 2)

Q) If the system of equations x – y = 1, ax + y = 2 has unique solution, then
A) a = 1
B) a = -1
C) a ≠ 1
D) a ≠ -1

Q) If α, β are the roots of x²– 1 = 0, then α+ β =
A) 0
B) 1
C) -1
D) 2

Q) For the equation 2019x+2020y = 4040 when x = 0 the value of y =
A) 2020
B) 2019
C) 4
D) 2

Q) Solution of the equations 7x + 5y = 12, 5x – 7y = -2 is not equal to
A)
B)
C)
D)

View Answer

A)
Explanation: 7x+ 5y = 12 ————(1)
5x – 7y = -2 —————–(2)
(1)x5-(2)x7
=> 35x + 25y = 60
35x – 49y = -14
– + –
______________
74y= 74 => y = 1
(1) => 7x + 5(1) = 12
=>7x + 5=12=>7x=7=>x=1
∴ x = 1, y = 1
∴ Solution of the given equation’s are not equal to option (1).

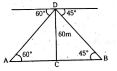
Q) From the top of the tower 60 mts high the angle of depression of two objects due north and due south of the tower are 60° and 45°, then the distance between two objects is
A) 60 √3 m
B) ( 60+20 √3)m
C) (60+ √3-1)m
D) (60+ √3+1)m

View Answer

B) ( 60+20 √3)m
Explanation: In △ACD,
Tan 60° = CD/AC
√3 = 60/AC

=> AC = 60/√3 = 20√3m
In △BCD,
Tan 45° = CD/BC
1 = 60/BC => BC = 60m
∴ AB = AC + BC = ( 20+√3 + 60)m

Q) What is the probability of getting an even number in a single throw of a die?
A)
B)
C)
D)

View Answer

A)
Explanation: S= {1,2,3, 4, 5, 6} => n (S) = 6
A = {2, 4, 6} => n(A) = 3
∴ P(A) = n(A)/n(S) = 3/6 = 1/2

Q) Find the mode when median is 125.6 and mean is 128.
A) 120
B) 120.8
C) 125
D) 128