TS Polycet (Polytechnic) 2020 Previous Question Paper with Answers And Model Papers With Complete Analysis

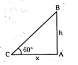
Q) If the angle of elevation of the Sun is 60°, then the ratio of a tree with its shadow.
A) 1 : 1
B) 1 : √3
C) √3 : 1
D) None of these

View Answer

C) √3 : 1
Explanation:
In △ABC,
Tan 60° = AB/AC
√3 = h/x
h/x = √3/1
h : x = √3 : 1

Q) Which of the following formula is associated to cylinder?
A)
B) π r²h
C)
D)

Q) If A, B, C, D are angles of a cyclic quadrilateral, then
sin A + sin B – sin C -sin D =
A) -1
B) 0
C) 1
D) 2

Q) cos 201° cos 202° cos 203° ………..cos 300° =
A)
B)
C)
D) 0

Q) To find out the slant height of a cone, we use ___________theorem.
A) Thales
B) S.A.S.
C) Pythagorus
D) S.S.S.

Q) If a cosθ+ b sinθ = p, a sinθ – b cosθ = q, then
A) a² + b² = p² + q²
B) a² + b² = p² – q²
C) a² – b² = p² + q²
D) a² – b² = p² – q²

View Answer

A) a² + b² = p² + q²
Explanation: a cosθ + b sinθ = p
a sinθ – b cosθ = q
(1)² + (2)²
a²cos2θ + b²sin²θ + 2ab sinθ cosθ = p²
= p² + q^{2
a2 (1) + b2 (1) = p2 + q2
a2 + b2 = p2 + q2}

Q) 1 radian =
A) 56°18’
B) 57°16’
C) 56°15’
D) 45°40’

Q) The radius of the sphere is increased by 100%, the volume of the resulting sphere is increased by
A) 200%
B) 700%
C) 500%
D) 900%

Q) △ABC ~△ XYZ; ∠C= 60°; ∠B = 75°, then ∠Z =_______________.
A) 90°
B) 75°
C) 45°
D) 60°

View Answer

D) 60°
Explanation: △ABC-△XYZ,
∠C = 60°, ∠B = 75°

∠A+∠B+∠C = 180°
∠A + 135° = 180°
∠A = 180° – 135°
∠A = 45°
∠C = ∠Z = 60°

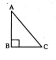
Q) In △ABC; BC² + AB² = AC², then _________ is the right angle
A) B
B) A
C) C
D) Can’t say

View Answer

A) B
Explanation: BC²+AB² = AC²

∠B = 90°